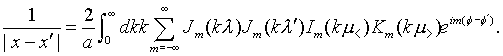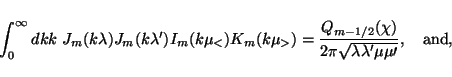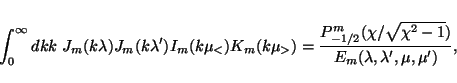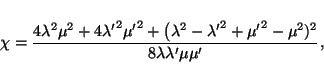Next: Two Fourth-Order Rotational Laplace
Up: Three Second-Order Rotational Laplace
Previous: Oblate Spheroidal Coordinates
Please feel free to enjoy my contributions to these interesting fields:
Numerical solution for 3D Poisson equation in circular cylindrical coordinates : Cohl et. al. (
1997,
1999a)
Green's function for 3D Laplace equation : Cohl et. al. (
1999a,
1999b,
2000,
2001,
2002)
Gravitational potential : Cohl et. al. (
1999a,
1999b,
2000,
2001,
2002)
Quadrics & cyclides : Cohl et. al. (
1999a,
1999b,
2000,
2001,
2002)
Heine identity : Cohl et. al. (
1999a,
1999b,
2000,
2001,
2002)
Cylindrical, toroidal, oblate and prolate spheroidal, parabolic, bispherical coordinate systems : Cohl et. al.
(1999a, 2000)
New addition theorems for rotationally invariant coordinate systems which R-separate 3D Laplace equation: Cohl et. al.
(1999a,
2000)
3D biharmonic, 3D triharmonic, and 3D higher harmonic Green's functions : Cohl (2002)
Spherical coordinate system : Cohl et. al.
(2001)
Coulomb direct (classical) and exchange (quantum) integrals/interactions : Cohl et. al.
(2001)
Two-electron interactions : Cohl et. al.
(2001)
Spherical azimuthal and separation angle Fourier expansions : Cohl et. al.
(2001)
Magnetic field of an infinitesimally thin circular current loop : Cohl & Tohline
(1999)
Symmetry properties of associated Legendre/toroidal functions : Cohl et. al.
(2000)
Whipple formulae for toroidal/associated Legendre functions : Cohl et. al.
(2000)
New addition theorem for spherical coordinates : Cohl et. al.
(2001)
Solar White Light Flares : Neidig et. al.
(1993)
1997 - Cohl, H. S., Xian-He Sun and J. E. Tohline
"Parallel Implementation of a Data-Transpose Technique
for the Solution of Poisson's Equation in Cylindrical Coordinates"
Proceedings of the 8th SIAM Conference on Parallel Processing
for Scientific Computing, Minneapolis, Minnesota, March.
1999a - Cohl, H. S.
"On the numerical solution of the cylindrical Poisson
equation for isolated self-gravitating systems"
The Louisiana State University
and Agricultural and Mechanical College, 122 pages
1999b - Cohl, H. S. and J. E. Tohline
"A Compact Cylindrical Green's Function
Expansion for the Solution of Potential Problems"
The Astrophysical Journal, 527, 86-101.
2000 - Cohl, H. S., J. E. Tohline, A. R. P. Rau, H. M. Srivastava
"Developments in determining the gravitational potential using toroidal functions"
Astronomische Nachrichten, 321, 5/6, 363-372.
2001 - Cohl, H. S., Rau, A. R. P., Tohline, J. E., Browne, D. A., Cazes, J. E. and Barnes, E. I.
"Useful alternative to the multipole expansion of 1/r potentials"
Physical Review A: Atomic and Molecular Physics and Dynamics, 64, 5, 52509.
2002 - Cohl, H. S.
"Portent of Heine's Reciprocal Square Root Identity"
Proceedings of the 3D Stellar Evolution Workshop, ed. R. Cavallo,
S. Keller, S. Turcotte, Livermore, California
CLICK TEXT TO READ PDF COPY OF PAPER
In parabolic coordinates --
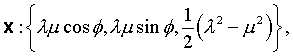 where
where
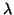 goes from 0 to
goes from 0 to 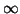 ,
,
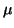 goes from
0 to
goes from
0 to 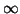 ,
and
,
and 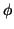 goes from 0 to
goes from 0 to 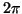 -- the surfaces
-- the surfaces
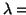 constant and
constant and
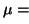 constant are both
paraboloids of revolution or parabolic bowls. The Green's function can be written as follows
(eq. [10.3.68] in Morse & Feshbach 1953):
constant are both
paraboloids of revolution or parabolic bowls. The Green's function can be written as follows
(eq. [10.3.68] in Morse & Feshbach 1953):
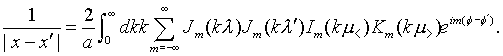 |
(40) |
Consequently, the following two expressions must be valid
definite integrals:
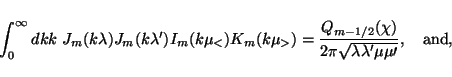 |
(41) |
and
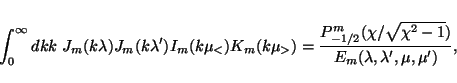 |
(42) |
where
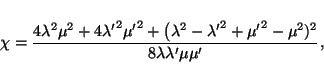 |
(43) |
and,
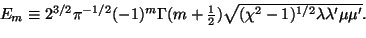
Howard S. Cohl
2000-10-05
Cohl, H. S., J. E. Tohline, A. R. P. Rau, H. M. Srivastava (2000)
Site at a Glance:
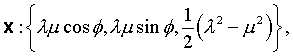 where
where