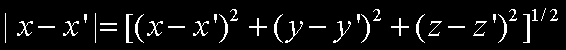Rotational Invariance in Other PDE's
Rotational invariance in the other PDE's of mathematical physics...
Helmholtz, Diffusion, Wave, Schroedinger, ...
The PDE's of Mathematical Physics
A new description of linear symmetries must proceed through an exhaustive
analytical survey of "new" symmetries present within the integrable
solutions of the linear PDE's of mathematical physics.
Inhomogeneous Laplace equation
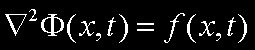
Inhomogeneous Helmholtz equation

Inhomogeneous Diffusion/Heat/Schrödinger/Euler-Poisson-Darboux equations
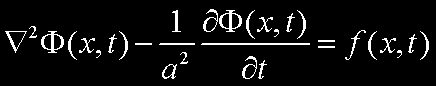
Inhomogeneous Wave equation
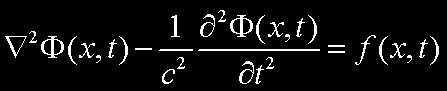
Biharmonic equation
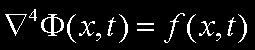
The new symmetries govern fundamental behavior in physics, mathematics,
engineering, chemistry, "biology", quantum mechanics,
general relativity, etc.
Green's Functions of Mathematical Physics
The infinite domain
Green's functions for the Linear PDE's of mathematical physics.
(from now on out the infinite-extent Green's function
is referred to as Green's function)
The Green's function for the 3D Laplace equation:
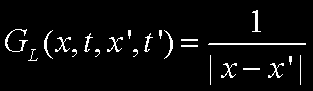
The Green's function for the 3D Wave equation:
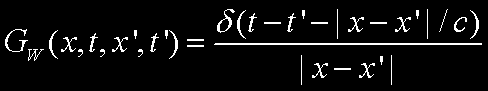
The Green's function for the 3D Helmholtz equation:
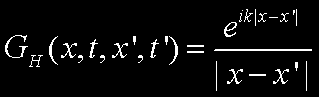
The Green's function for the 3D Heat/Diffusion/Schroedinger/EPD equation:
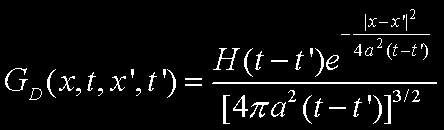
The Green's function for the 3D Biharmonic equation:
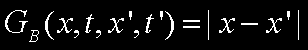
where
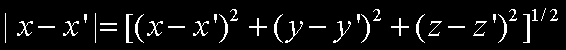
next page
The PDE's of Mathematical Physics
Inhomogeneous Laplace equation
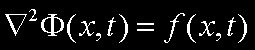
Inhomogeneous Helmholtz equation

Inhomogeneous Diffusion/Heat/Schrödinger/Euler-Poisson-Darboux equations
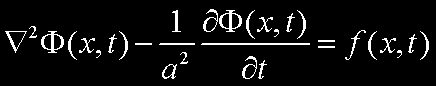
Inhomogeneous Wave equation
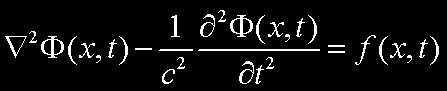
Biharmonic equation
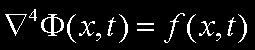
The new symmetries govern fundamental behavior in physics, mathematics, engineering, chemistry, "biology", quantum mechanics,
general relativity, etc.
Green's Functions of Mathematical Physics
(from now on out the infinite-extent Green's function is referred to as Green's function)
The Green's function for the 3D Laplace equation:
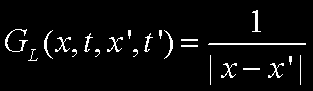
The Green's function for the 3D Wave equation:
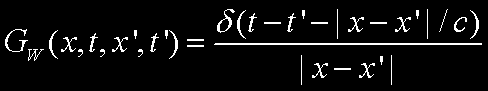
The Green's function for the 3D Helmholtz equation:
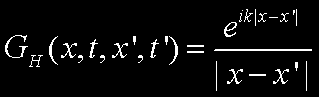
The Green's function for the 3D Heat/Diffusion/Schroedinger/EPD equation:
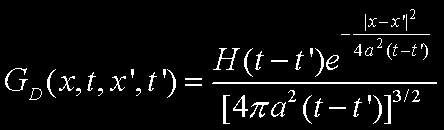
The Green's function for the 3D Biharmonic equation:
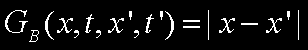
where